Remember our friend E = IR? Well that equation works perfectly well for DC
circuits, those most commonly found in electronics. However, that simple
equation doesn’t actually suffice for AC circuits. Both frequency and phase come
into play when computing values in AC circuits.

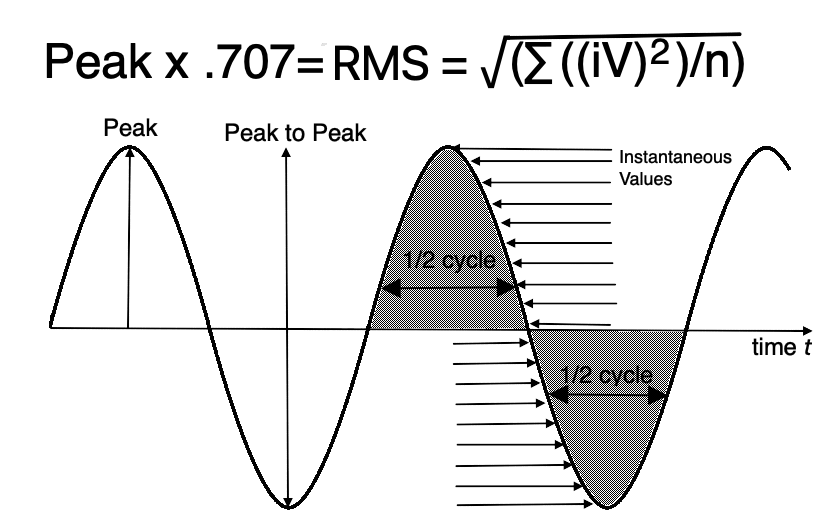
If we take the value of the peak of an AC waveform and multiply it by 0.707 we
will have the RMS or effective value of the waveform. The RMS value is the
portion of the AC waveform that can do actual work (the portion of the drawing
that is grayed out). RMS means root-mean-square. It is the square root of the
average (or mean) of the sum of the squares of the instantaneous values of the
voltage or current.

The RMS value of an AC waveform is equivalent to the peak value of a DC
waveform. DC waveforms don’t really have peaks, it is a constant value. To
determine the value of AC that is equivalent to DC, we need to multiply the AC
effective value by 1.414 (the reciprocal of .707 or 1/.707).

When problems are given to be solved, it is presumed that the effective value is
to be employed. For instance, when the power company states that it is supplying
120VAC it means 120 Veff which is 120 x 1.414 or about 170V peak.

73… W3SEH